
math notes for class 12 three chapter 11: dimensional geometry download pdf , three dimensional geometry 12 notes, class 12 maths notes, three dimensional geometry class 12, three dimensional geometry class 12 notes, class 12 three dimensional geometry, note maths, maths notes, three dimensional geometry, class 12 cmaths chapter 11 notes, 12th standard maths notes, 12th std maths notes, class 12 maths notes chapter 11, three dimensional geometry chapter class 12 notes
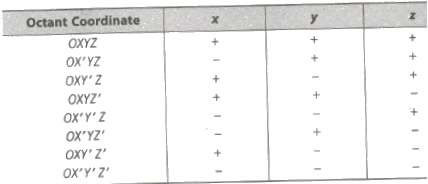
The three mutually perpendicular lines in a space which divides the space into eight parts and if these perpendicular lines are the coordinate axes, then it is said to be a coordinate system.

Let P(x1, y1, z1) and Q(x2, y2, z2) be two given points. The distance between these points is
given by PQ √(x2 – x1)2 + (y2 – y1)2 + (z2 – z1)2
The distance of a point P(x, y, z) from origin O is OP = √x2 + y2 + z2
(i) The coordinates of any point, which divides the join of points P(x1, y1, z1) and Q(x2, y2, z2)
in the ratio m : n internally are (mx2 + nx1 / m + n, my2 + ny1 / m + n, mz2 + nz1 / m + n)
(ii) The coordinates of any point, which divides the join of points P(x1, y1, z1) and Q(x2, y2, z2)
in the ratio m : n externally are (mx2 – nx1 / m – n, my2 – ny1 / m – n, mz2 – nz1 / m – n)
(iii) The coordinates of mid-point of P and Q are (x1 + x2 / 2 , y1 + y2 / 2, z1 + z2 / 2)
(iv) Coordinates of the centroid of a triangle formed with vertices P(x1, y1, z1) and Q(x2, y2, z2)
and R(x3, y3, z3) are (x1 + x2 + x3 / 3 , y1 + y2 + y3 / 3, z1 + z2 + z3 / 3)
(v) Centroid of a Tetrahedron
If (x1, y1, z1), (x2, y2, z2), (x3, y3, z3) and (x4, y4, z4) are the vertices of a tetrahedron, then its centroid G is given by
(x1 + x2 + x3 + x4 / 4 , y1 + y2 + y3 + y4 / 4, z1 + z2 + z3 + z4 / 4)
If a directed line segment OP makes angle α, β and γ with OX , OY and OZ respectively, then
Cos α, cos β and cos γ are called direction cosines of up and it is represented by l, m, n.
i.e.,
l = cos α
m = cos β and n = cos γ
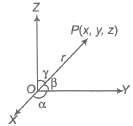
If OP = r, then coordinates of OP are (lr, mr , nr)
(i) If 1, m, n are direction cosines of a vector r, then
(a) r = |r| (li + mj + nk) ⇒ r = li + mj + nk
(b) l2 + m2 + n2 = 1
(c) Projections of r on the coordinate axes are
(d) |r| = l|r|, m|r|, n|r| / √sum of the squares of projections of r on the coordinate axes
(ii) If P(x1, y1, z1) and Q(x2, y2, z2) are two points, such that the direction cosines of PQ are l,
m, n. Then, x2 – x1 = l|PQ|, y2 – y1 = m|PQ|, z2 – z1 = n|PQ| These are projections of PQ on X , Y and Z axes, respectively.
(iii) If 1, m, n are direction cosines of a vector r and a b, c are three numbers, such that l / a = m
/ b = n / c.
Then, we say that the direction ratio of r are proportional to a, b, Also, we have l = a / √a2 + b2 + c2, m = b / √a2 + b2 + c2, n = c / √a2 + b2 + c2
(iv) If θ is the angle between two lines having direction cosines l1, m1, n1 and 12, m2, n2, then cos θ = l112 + m1m2 + n1n2
(a) Lines are parallel, if l1 / 12 = m1 / m2 = n1 / n2
(b) Lines are perpendicular, if l112 + m1m2 + n1n2
(v) If θ is the angle between two lines whose direction ratios are proportional to a1, b1, c1 and
a2, b2, c2 respectively, then the angle θ between them is given by cos θ = a1a2 + b1b2 + c1c2 / √a2
1 + b2
1 + c2
1 √a2
2 + b2
2 + c2
2
Lines are parallel, if a1 / a2 = b1 / b2 = c1 / c2
Lines are perpendicular, if a1a2 + b1b2 + c1c2 = 0.
(vi) The projection of the line segment joining points P(x1, y1, z1) and Q(x2, y2, z2) to the line
having direction cosines 1, m, n is |(x2 – x1)l + (y2 – y1)m + (z2 – z1)n|.
(vii) The direction ratio of the line passing through points P(x1, y1, z1) and Q(x2, y2, z2) are proportional to x2 – x1, y2 – y1 – z2 – z1
Then, direction cosines of PQ arex2 – x1 / |PQ|, y2 – y1 / |PQ|, z2 – z1 / |PQ|
If the vertices of a triangle be A(x1, y1, z1) and B(x2, y2, z2) and C(x3, y3, z3), then
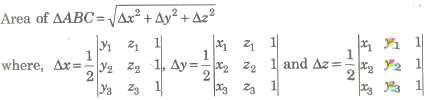
If l(x1, m1, n1) and l(x2, m2, n2) be the direction cosines of two given lines, then the angle θ between them is given by cos θ = l112 + m1m2 + n1n2
(i) The angle between any two diagonals of a cube is cos-1 (1 / 3).
(ii) The angle between a diagonal of a cube and the diagonal of a face (of the cube is cos-1 (√2 /
3)
The two equations of the line ax + by + cz + d = 0 and a’ x + b’ y + c’ z + d’ = 0 together represents a straight line.
1. Equation of a straight line passing through a fixed point A(x1, y1, z1) and having direction ratios a, b, c is given by x – x1 / a = y – y1 / b = z – z1 / c, it is also called the symmetrically form of a line.
Any point P on this line may be taken as (x1 + λa, y1 + λb, z1 + λc), where λ ∈ R is parameter.
If a, b, c are replaced by direction cosines 1, m, n, then λ, represents
distance of the point P
from the fixed point A.
2. Equation of a straight line joining two fixed points A(x1, y1, z1) and B(x2, y2, z2) is given by x – x1 / x2 – x1 = y – y1 / y2 – y1 = z – z1 / z2 – z1
3. Vector equation of a line passing through a point with position vector a and parallel to vector
b is r = a + λ b, where A, is a parameter.
4. Vector equation of a line passing through two given points having position vectors a and b is
r = a + λ (b – a) , where λ is a parameter.
5. (a) The length of the perpendicular from a point ![]() on the line r – a + λ b is given by
on the line r – a + λ b is given by
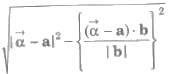
(b) The length of the perpendicular from a point P(x1, y1, z1) on the line
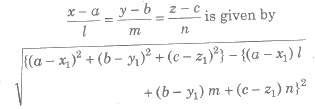
where, 1, m, n are direction cosines of the line.
6. Skew Lines Two straight lines in space are said to be skew lines, if they are neither parallel
nor intersecting.
7. Shortest Distance If l1 and l2 are two skew lines, then a line perpendicular to each of lines 4 and 12 is known as the line of shortest distance.
If the line of shortest distance intersects the lines l1 and l2 at P and Q respectively, then the distance PQ between points P and Q is known as the shortest distance between l1 and l2.
8. The shortest distance between the lines
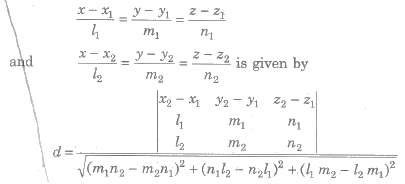
9. The shortest distance between lines r = a1 + λb1 and r = a2 + μb2 is given by

10. The shortest distance parallel lines r = a1 + λb1 and r = a2 + μb2 is given by
![]()
11. Lines r = a1 + λb1 and r = a2 + μb2 are intersecting lines, if (b1 * b2) * (a2 – a1) = 0.
12. The image or reflection (x, y, z) of a point (x1, y1, z1) in a plane ax + by + cz + d = 0 is given by x – x1 / a = y – y1 / b = z – z1 / c = – 2 (ax1 + by1 + cz1 + d) / a2 + b2 + c2
13. The foot (x, y, z) of a point (x1, y1, z1) in a plane ax + by + cz + d = 0 is given by x – x1 / a = y – y1 / b = z – z1 / c = – (ax1 + by1 + cz1 + d) / a2 + b2 + c2
14. Since, x, y and z-axes pass through the origin and have direction cosines (1, 0, 0), (0, 1, 0)
and (0, 0, 1), respectively. Therefore, their equations are
x – axis : x – 0 / 1 = y – 0 / 0 = z – 0 / 0
y – axis : x – 0 / 0 = y – 0 / 1 = z – 0 / 0z – axis : x – 0 / 0 = y – 0 / 0 = z – 0 / 1
A plane is a surface such that, if two points are taken on it, a straight line joining them lies wholly in the surface.
The general equation of the first degree in x, y, z always represents a plane. Hence, the general equation of the plane is ax + by + cz + d = 0. The coefficient of x, y and z in the cartesian equation of a plane are the direction ratios of normal to the plane.
The equation of a plane passing through a given point (x1, y1, z1) is given by a(x – x1) + b (y — y1) + c (z — z1) = 0.
(i) The equation of a plane, which is at a distance p from origin and the direction cosines of the
normal from the origin to the plane are l, m, n is given by lx + my + nz = p.
(ii) The coordinates of foot of perpendicular N from the origin on the plane are (1p, mp, np).
The intercept form of equation of plane represented in the form of x / a + y / b + z / c = 1
where, a, b and c are intercepts on X, Y and Z-axes, respectively.
For x intercept Put y = 0, z = 0 in the equation of the plane and obtain the value of x. Similarly, we can determine for other intercepts.
(i) Equation of a plane passing through the point A(x1, y1, z1) and parallel to two given lines with direction ratios
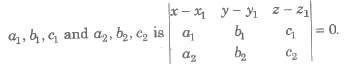
(ii) Equation of a plane through two points A(x1, y1, z1) and B(x2, y2, z2) and parallel to a line with direction ratios a, b, c is
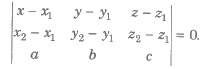
(iii) The Equation of a plane passing through three points A(x1, y1, z1), B(x2, y2, z2) and C(x3, y3, z3) is
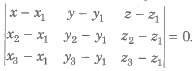
(iv) Four points A(x1, y1, z1), B(x2, y2, z2), C(x3, y3, z3) and D(x4, y4, z4) are coplanar if and only if

(v) Equation of the plane containing two coplanar lines

The angle between two planes is defined as the angle between the normal to them from any point.
Thus, the angle between the two planes a1x + b1y + c1z + d1 = 0 and a2x + b2y + c2z + d2 = 0
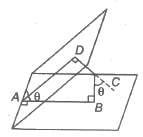
is equal to the angle between the normals with direction cosines ± a1 / √Σ a2
1, ± b1 / √Σ a2
1, ± c1 / √Σ a2
1 and ± a2 / √Σ a2
2, ± b2 / √Σ a2
2, ± c2 / √Σ a2
2
If θ is the angle between the normals, then cos θ = ± a1a2 + b1b2 + c1c2 / √a21 + b2 1 + c2 1 √a2 2 + b2 2 + c2 2
Two planes are parallel or perpendicular according as the normals to them are parallel or perpendicular.
Hence, the planes a1x + b1y + c1z + d1 = 0 and a2x + b2y + c2z + d2 = 0 are parallel, if a1 / a2 = b1 / b2 = c1 / c2 and perpendicular, if a1a2 + b1b2 + c1c2 = 0.
Note The equation of plane parallel to a given plane ax + by + cz + d = 0 is given by ax + by +
cz + k = 0, where k may be determined from given conditions.
In Vector Form The angle between a line r = a + λ b and plane r *• n = d, is defined as the complement of the angle between the line and normal to the plane:
sin θ = n * b / |n||b|
In Cartesian Form The angle between a line x – x1 / a1 = y – y1 / b1 = z – z1 / c1 and plane a2x + b2y + c2z + d2 = 0 is sin θ = a1a2 + b1b2 + c1c / √a2 1 + b2 1 + c2 1 √a22 + b2 2 + c2 2
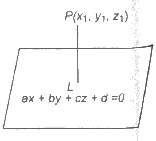
Let the plane in the general form be ax + by + cz + d = 0. The distance of the point P(x1, y1, z1) from the plane is equal to
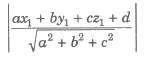
If the plane is given in, normal form lx + my + nz = p. Then, the distance of the point P(x1, y1, z1) from the plane is |lx1 + my1 + nz1 – p|.
If ax + by + cz + d1 = 0 and ax + by + cz + d2 = 0 be equation of two parallel planes. Then, the distance between them is
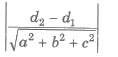
The bisector planes of the angles between the planes a1x + b1y + c1z + d1 = 0, a2x + b2y + c2z + d2 = 0 is a1x + b1y + c1z + d1 / √Σa2 1 = ± a2x + b2y + c2z + d2 / √Σa2 2
One of these planes will bisect the acute angle and the other obtuse angle between the given plane.
A sphere is the locus of a point which moves in a space in such a way that its distance from a fixed point always remains constant.
The equation of the sphere with centre (a, b, c) and radius r is (x – a)2 + (y – b)2 + (z – c)2 = r2 …….(i)
In generally, we can write x2 + y2 + z2 + 2ux + 2vy + 2wz + d = 0
Here, its centre is (-u, v, w) and radius = √u2 + v2 + w2 – d
The vector equation of a sphere of radius a and Centre having position vector c is |r – c| = a
(i) The general equation of second degree in x, y, z is ax2 + by2 + cz2 + 2hxy + 2kyz + 2lzx +
2ux + 2vy + 2wz + d = 0 represents a sphere, if
(a) a = b = c (≠ 0)
(b) h = k = 1 = 0
The equation becomes ax2 + ay2 + az2 + 2ux + 2vy + 2wz + d – 0 …(A)
To find its centre and radius first we make the coefficients of x2, y2 and z2 each unity by
dividing throughout by a.
Thus, we have x2+y2+z2 + (2u / a) x + (2v / a) y + (2w / a) z + d / a = 0 …..(B)
∴ Centre is (- u / a, – v / a, – w / a)
and radius = √u2 / a2 + v2 / a2 + w2 / a2 – d / a
= √u2 + v2 + w2 – ad / |a| .
(ii) Any sphere concentric with the sphere x2 + y2 + z2 + 2ux + 2vy + 2wz + d = 0 is x2 + y2 + z2 + 2ux + 2vy + 2wz + k = 0
(iii) Since, r2 = u2 + v2 + w2 — d, therefore, the Eq. (B) represents a real sphere, if u2 +v2 + w2 — d > 0
(iv) The equation of a sphere on the line joining two points (x1, y1, z1) and (x2, y2, z2) as a
diameter is
(x – x1) (x – x1) + (y – y1) (y – y2) + (z – z1) (z – z2) = 0.
(v) The equation of a sphere passing through four non-coplanar points (x1, y1, z1), (x2, y2, z2),
(x3, y3, z3) and (x4, y4, z4) is

The plane lx + my + nz = p will touch the sphere x2 + y2 + z2 + 2ux + 2vy + 2 wz + d = 0, if length of the perpendicular from the centre ( – u, – v,— w)= radius, i.e., |lu – mv – nw – p| / √l2 + m2 + n2
= √u2 + v2 + w2 – d (lu – mv – nw – p)2 = (u2 + v2 + w2 – d) (l2 + m2 + n2)
Consider a sphere intersected by a plane. The set of points common to both sphere and plane is
called a plane section of a sphere. In ΔCNP, NP2 = CP2 – CN2 = r2 – p2
∴ NP = √r2 – p2
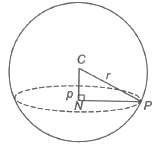
Hence, the locus of P is a circle whose centre is at the point N, the foot of the perpendicular
from the centre of the sphere to the plane.
The section of sphere by a plane through its centre is called a great circle. The centre and radius
of a great circle are the same as those of the sphere.
Copyright @ ncerthelp.com A free educational website for CBSE, ICSE and UP board.