
ncert math notes for class 12 chapter 1 relations and functions download pdf, relations and functions 12 notes, class 12 maths notes, relations and functions class 12, relations and functions class 12 notes, class 12 relations and functions, note maths, maths notes, relations and functions, class 12 cmaths chapter 1 notes, 12th standard maths notes, 12th std maths notes, class 12 maths notes chapter 1, relations and functions chapter class 12 notes
NCERT Notes Mathematics for Class 12
Chapter 1:- Relations and Functions
Let A and B be .two non-empty sets, then a function f from set A to set B is a rule whichassociates each element of A to a unique element of B.
It is represented as f: A → B and function is also called mapping.
f : A → B is called a real function, if A and B are subsets of R.
Domain and codomain of a function f is a set of all real numbers x for which f(x) is a real number. Here, set A is domain and set B is codomain.
Range of a real function, f is a set of values f(x) which it attains on the points of its domain.
Real functions are generally classified under two categories algebraic functions and transcendental functions.
Some algebraic functions are given below
(i) Polynomial Functions If a function y = f(x) is given by
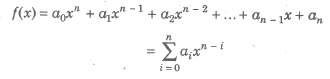
where, a0, a1, a2,…, an are real numbers and n is any non -negative integer, then f (x) is called a polynomial function in x.
If a0 ≠ 0, then the degree of the polynomial f(x) is n. The domain of a polynomial function is the set of real number R.
e.g., y = f(x) = 3x5 – 4x2 – 2x +1
is a polynomial of degree 5.
(ii) Rational Functions If a function y = f(x) is given by f(x) = φ(x) / Ψ(x)where, φ(x) and Ψ(x) are polynomial functions, then f(x) is called rational function in x.
(iii) Irrational Functions The algebraic functions containing one or more terms having nonintegral
rational power x are called irrational functions.
e.g., y = f(x) = 2√x –3√x + 6
A. function, which is not algebraic, is called a transcendental function. Trigonometric, Inverse trigonometric, Exponential, Logarithmic, etc are transcendental functions.
(i) Explicit Functions A function is said to be an explicit function, if it is expressed in the form y = f(x).
(ii) Implicit Functions A function is said to be an implicit function, if it is expressed in the
form f(x, y) = C, where C is constant.
e.g., sin (x + y) – cos (x + y) = 2
(i) The set of real numbers x, such that a ≤ x ≤ b is called a closed interval and denoted by [a, b] i.e., {x: x ∈ R, a ≤ x ≤ b}.
(ii) Set of real number x, such that a < x < b is called open interval and is denoted by (a, b) i.e., {x: x ∈ R, a < x < b}
(iii) Intervals [a,b) = {x: x ∈ R, a ≤ x ≤ b} and (a, b] = {x: x ≠ R, a < x ≤ b} are called semiopen and semi-closed intervals.
The function that associates to each real number x, this fixed number c is called a constant function i.e., y = f{x) = c for all x ∈ R.
Domain of f{x) = R
Range of f{x) = {c}

The function that associates to each real number x for the same number x, is called the identity function. i.e., y = f(x) = x, ∀ x ∈
R.
Domain of f(x) =
R
Range f(x) = R
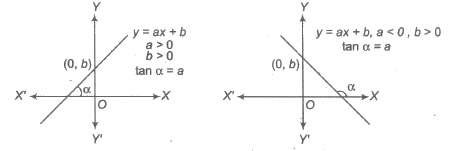
If a and b be fixed real numbers, then the linear function is defmed as y = f(x) = ax + b, where a and b are constants.
Domain of f(x) = R
Range of f(x) = R
The graph of a linear function is given in the following diagram, which is a straight line with slope a.
If a, b and c are fixed real numbers, then the quadratic function is expressed as y = f(x) = ax2 + bx + c, a ≠ 0 ⇒ y = a (x + b / 2a)2 + 4ac – b2 / 4a
which is equation of a parabola in downward, if a < 0 and upward, if a > 0 and vertex at ( – b / 2a, 4ac – b2 / 4a).
Domain of f(x) = R
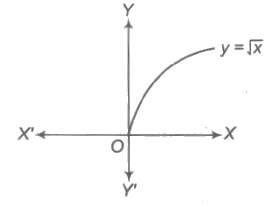
Range of f(x) is [ – ∞, 4ac – b2 / 4a], if a < 0 and [4ac – b2 / 4a, ∞], if a > 0 5. Square Root Function Square root function is defined by y = F(x) = √x, x ≥ 0.

Square root function is defined by y = F(x) = √x, x ≥ 0.
Domain of f(x) = [0, ∞)
Range of f(x) = [0, ∞)
Exponential function is given by y = f(x) = ax, where a > 0, a ≠ 1.
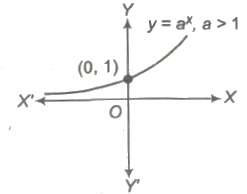
A logarithmic function may be given by y = f(x) = loga x, where a > 0, a ≠ 1 and x > 0.
The graph of the function is as shown below. which is increasing, if a > 1 and decreasing, if 0 < a < 1.
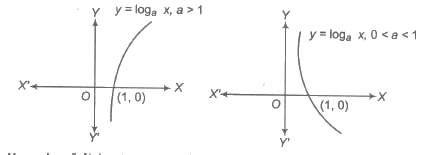
Domain of f(x) = (0, ∞)
Range of f(x) = R
The power function is given by y = f(x) = xn ,n ∈ I,n≠ 1, 0. The domain and range of the graph y = f(x), is depend on n.
(a) If n is positive even integer.
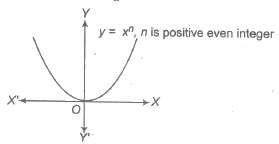
i.e., f(x) = x2, x4 ,….
Domain of f(x) = R
Range of f(x) = [0, ∞)
(b) If n is positive odd integer.
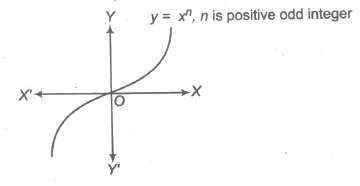
i.e., f(x) = x3, x5 ,….
Domain of f(x) = R
Range of f(x) = R
(c) If n is negative even integer.
i.e., f(x) = x- 2, x – 4 ,….
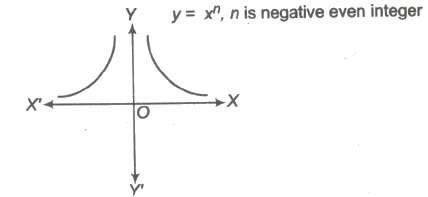
Domain of f(x) = R – {0}
Range of f(x) = (0, ∞)
(d) If n is negative odd integer.
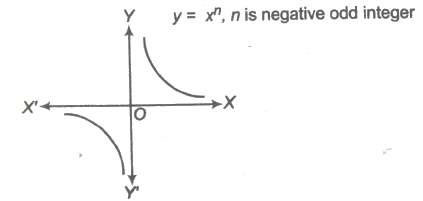
i.e., f(x) = x- 1, x – 3 ,….
Domain of f(x) = R – {0}
Range of f(x) = R – {0}
Modulus function is given by y = f(x) = |x| , where |x| denotes the absolute value of x, that is
|x| = {x, if x ≥ 0, – x, if x < 0
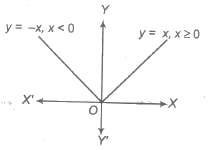
Domain of f(x) = R
Range of f(x) = [0, &infi;)
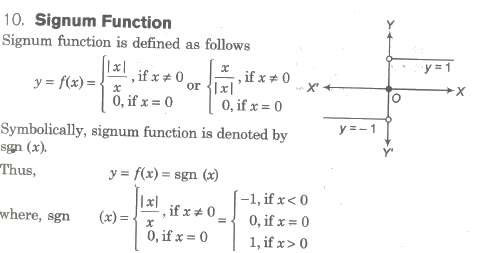
Domain of f(x) = R
Range of f(x) = {-1, 0, 1}
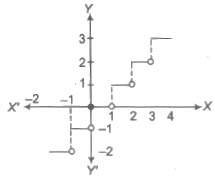
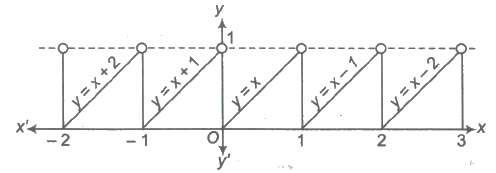
The greatest integer function is defined as y = f(x) = [x]
where, [x] represents the greatest integer less than or equal to x. i.e., for any integer n, [x] = n, if n ≤ x < n + 1 Domain of f(x) = R Range of f(x) = I
(i) [x + n] = n + [x], n ∈ I
(ii) x = [x] + {x}, {x} denotes the fractional part of x.
(iii) [- x] = – [x], -x ∈ I
(iv) [- x] = – [x] – 1, x ∈ I
(v) [x] ≥ n ⇒ x ≥ n,n ∈ I
(vi) [x] > n ⇒ x ⇒ n+1, n ∈ I
(vii) [x] ≤ n ⇒ x < n + 1, n ∈ I
(viii) [x] < n ⇒ x < n, n ∈ I
(ix) [x + y] = [x] + [y + x – [x}] for all x, y ∈ R
(x) [x + y] ≥ [x] + [y]
(xi) [x] + [x + 1 / n] + [x + 2 / n] +…+ [x + n – 1 / n] = [nx], n ∈ N
The least integer function which is greater than or equal to x and it is denoted by (x). Thus, (3.578) = 4, (0.87) = 1, (4) = 4, (- 8.239) = – 8, (- 0.7) = 0
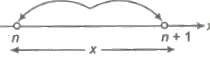
In general, if n is an integer and x is any real number between n and (n + 1).
i.e., n < x ≤ n + 1, then (x) = n + 1
∴ f(x) = (x)
Domain of f = R
Range of f= [x] + 1
It is denoted as f(x) = {x} and defined as
(i) {x} = f, if x = n + f, where n ∈ I and 0 ≤ f < 1
(ii) {x} = x – [x]
i.e., {O.7} = 0.7, {3} = 0, { – 3.6} = 0.4
(iii) {x} = x, if 0 ≤ x ≤ 1
(iv) {x} = 0, if x ∈ I
(v) { – x} = 1 – {x}, if x ≠ I
(i) Domain = R
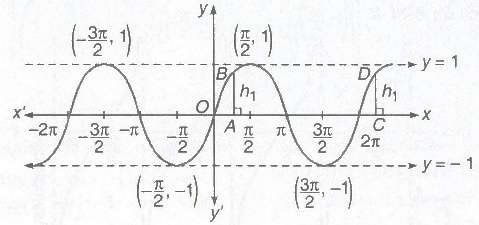
(ii) Range = [-1,1]
(iii) Period = 2π
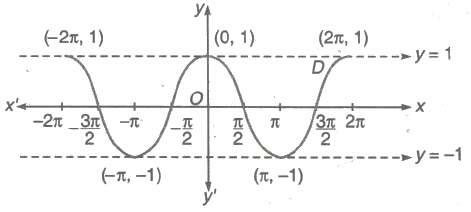
(i) Domain = R
(ii) Range = [-1,1]
(iii) Period = 2π
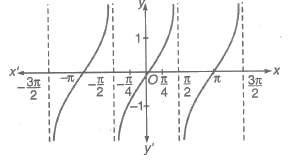
(i) Domain = R ~ (2n + 1) π / 2, n ∈ I
(ii) Range = [- &infi;, &infi;]
(iii) Period = π
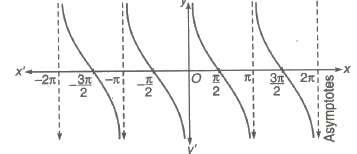
(i) Domain = R ~ nπ, n ∈ I
(ii) Range = [- &infi;, &infi;]
(iii) Period = π
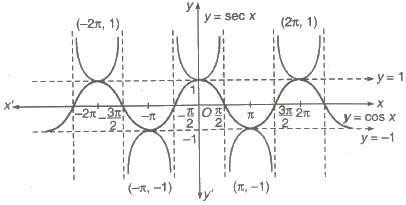
(i) Domain = R ~ (2n + 1) π / 2, n ∈ I
(ii) Range = [- &infi;, 1] ∪ [1, &infi;)
(iii) Period = 2π

(i) Domain = R ~ nπ, n ∈ I
(ii) Range = [- &infi;, – 1] ∪ [1, &infi;)
(iii) Period = 2π
Let f: x → R and g : X → R be two real functions, then
(i) Sum The sum of the functions f and g is defined as f + g : X → R such that (f + g) (x) = f(x) + g(x).
(ii) Product The product of the functions f and g is defined as
fg : X → R, such that (fg) (x) = f(x) g(x)
Clearly, f + g and fg are defined only, if f and g have the same domain. In case, the domain of f
and g are different. Then, Domain of f + g or fg = Domain of f ∩ Domain of g.
(iii) Multiplication by a Number Let f : X → R be a function and let e be a real number .
Then, we define cf: X → R, such that (cf) (x) = cf (x), ∀ x ∈ X.
(iv) Composition (Function of Function) Let f : A → B and g : B → C be two functions.
We define gof : A → C, such that
got (c) = g(f(x)), ∀ x ∈ A
Alternate There exists Y ∈ B, such that if f(x) = y and g(y) = z, then got (x) = z
(i) Put f(T + x) = f(x) and solve this equation to find the positive values of T independent of x.
(ii) If no positive value of T independent of x is obtained, then f(x) is a non-periodic function.
(iii) If positive val~es ofT independent of x are obtained, then f(x) is a periodic function and the least positive value of T is the period of the function f(x).
(i) Constant function is periodic with no fundamental period.
(ii) If f(x) is periodic with period T, then 1 / f(x) and. √f(x) are also periodic with f(x) same period T.
{iii} If f(x) is periodic with period T1 and g(x) is periodic with period T2, then f(x) + g(x) is periodic with period equal to LCM of T1 and T2, provided there is no positive k, such that f(k +
x) = g(x) and g(k + x) = f(x).
(iv) If f(x) is periodic with period T, then kf (ax + b) is periodic with period T / |a|’ where a, b ,k ∈ R and a, k ≠ 0.
(v) sin x, cos x, sec x and cosec x are periodic functions with period 2π.
(vi) tan x and cot x are periodic functions with period π.
(vii) |sin x|, |cos x|, |tan x|, |cot x|, |sec x| and |cosec x| are periodic functions with period π.
(viii) sinn x, cosn x, secn x and cosecnx are periodic functions with period 2π when n is odd, or π
when n is even .
(ix) tann x and cotnx are periodic functions with period π.
(x) |sin x| + |cos x|, |tan x| + |cot x| and |sec x| + |cosec x| are periodic with period π / 2.
Even Functions A real function f(x) is an even function, if f( -x) = f(x).
Odd Functions A real function f(x) is an odd function, if f( -x) = – f(x).
(i) Even function ± Even function = Even function.
(ii) Odd function ± Odd function = Odd function.
(iii) Even function * Odd function = Odd function.
(iv) Even function * Even function = Even function.
(v) Odd function * Odd function = Even function.
(vi) gof or fog is even, if anyone of f and g or both are even.
(vii) gof or fog is odd, if both of f and g are odd.
(viii) If f(x) is an even function, then d / dx f(x) or ∫ f(x) dx is odd and if dx .. f(x) is an odd
function, then d / dx f(x) or ∫ f(x) dx is even.
(ix) The graph of an even function is symmetrical about Y-axis.
(x) The graph of an odd function is symmetrical about origin or symmetrical in opposite quadrants.
(xi) An even function can never be one-one, however an odd function mayor may not be oneone.
The mapping f: A → B is a called one-one function, if different elements in A have different images in B. Such a mapping is known as injective function or an injection.
(i) Analytically If x1, x2 ∈ A,
then f(x1) = f(x2) => x1 = x2 or equivalently x1 ≠ x2 => f(x1) ≠ f(x2)
(ii) Graphically If any .line parallel to x-axis cuts the graph of the function atmost at one point,
then the function is one-one.
(iii) Monotonically Any function, which is entirely increasing or decreasing in whole domain,
then f(x) is one-one.
Number of One-One Functions Let f : A → B be a function, such that A and B are finite sets
having m and n elements respectively, (where, n > m).
The number of one-one functions
n(n – 1)(n – 2) …(n – m + 1) = { nPm, n ≥ m, 0, n < m
The function f : A → B is called many – one function, if two or more than two different
elements in A have the same image in B.
If the function f: A → B is such that each element in B (codomain) is the image of atleast one
element of A, then we say that f is a function of A ‘onto’ B.
Thus, f: A → B, such that f(A) =
i.e., Range = Codomain
Note Every polynomial function f: R → R of degree odd is onto.
Number of Onto (surjective) Functions Let A and B are finite sets having m and n elements
respectively, such that 1 ≤ n ≤ m, then number of onto (surjective) functions from A to B is nΣr = 1 (- 1)n – r nCr rm = Coefficient ofn in n! (ex – 1)r If f : A → B is such that there exists atleast one element in codomain which is not the image of
Thus, f : A → B, such that f(A) ⊂ B
i.e., Range ⊂ Codomain
(i) If f and g are injective, then fog and gof are injective.
(ii) If f and g are surjective, then fog is surjective.
(iii) Iff and g are bijective, then fog is bijective.
Let f : A → B is a bijective function, i.e., it is one-one and onto function.

We define g : B → A, such that f(x) = y => g(y) = x, g is called inverse of f and vice-versa.
Symbolically, we write g = f-1
Thus, f(x) = y => f-1(y) = x
Copyright @ ncerthelp.com A free educational website for CBSE, ICSE and UP board.