Class 12 Maths NCERT Solutions For Chapter 5 Continuity and Differentiability

Chapter 5 Continuity and Differentiability

Download NCERT Solutions for Class 12 Mathematics

(Link of Pdf file is given below at the end of the Questions List)

In this pdf file you can see answers of following Questions

EXERCISE 5.1

Question 1. Prove that the function f (x) = 5x – 3 is continuous at x = 0, at x = – 3 and at x = 5.

QuestioQuestion Examine the continuity of the function f (x) = 2x2 – 1 at x = 3.

Question 3. Examine the following functions for continuity.
(a) f (x) = x – 5
(b) f (x) = 1 x − 5
(c) f (x) = 2 25 5 x x − +
(d) f (x) = | x – 5 |

Question 4. Prove that the function f (x) = xn is continuous at x = n, where n is a positive integer.

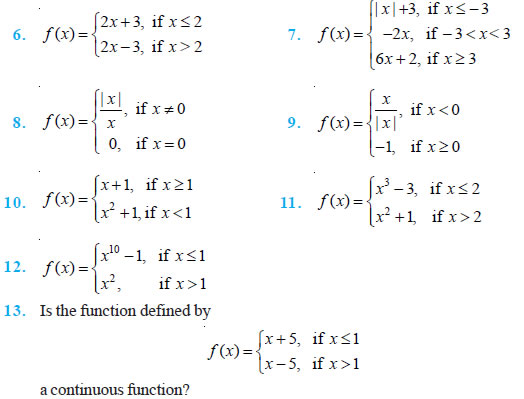
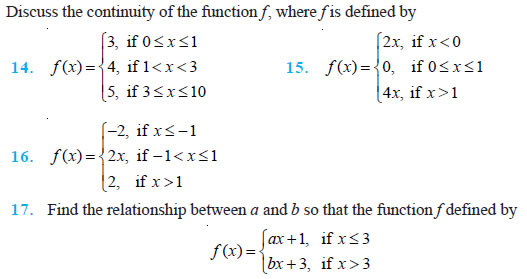
Question 5. Is the function f defined by , if 1 ( ) 5, if > 1 x x f x x ≤ = continuous at x = 0? At x = 1? At x = 2? Find all points of discontinuity of f, where f is defined by

Question 18. For what value of λ is the function defined by ( 2 2 ), if 0 ( ) 4 1, if 0 f x x x x x x ⎧⎪λ − ≤ = + > continuous at x = 0? What about continuity at x = 1?

Question 19. Show that the function defined by g (x) = x – [x] is discontinuous at all integral points. Here [x] denotes the greatest integer less than or equal to x.

Question 20. Is the function defined by f (x) = x2 – sin x + 5 continuous at x = π?

Question 21. Discuss the continuity of the following functions:
(a) f (x) = sin x + cos x
(b) f (x) = sin x – cos x
(c) f (x) = sin x . cos x

Question 22. Discuss the continuity of the cosine, cosecant, secant and cotangent functions.

Question 23. Find all points of discontinuity of f, where sin , if 0 ( ) 1, if 0 x x f x x x x < = + ≥

Question 24. Determine if f defined by 2 sin 1 , if 0 ( ) 0, if 0 x x f x x x ≠ = = is a continuous function?

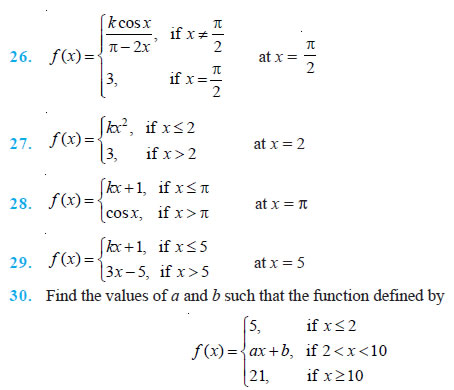
is a continuous function.

Question 31. Show that the function defined by f (x) = cos (x2) is a continuous function.

Question 32. Show that the function defined by f (x) = | cos x | is a continuous function.

Question 33. Examine that sin | x | is a continuous function.

Question 34. Find all the points of discontinuity of f defined by f (x) = | x | – | x + 1 |.

EXERCISE 5.2

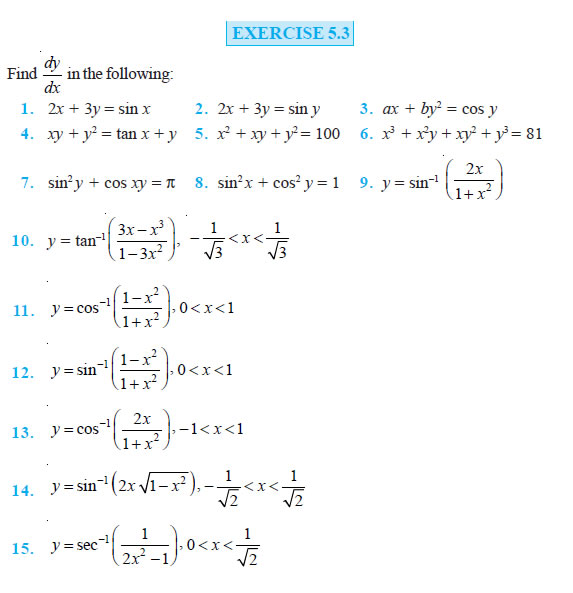
Differentiate the functions with respect to x in Exercises 1 to 8.

Question 1. sin (x2 + 5)

Question 2. cos (sin x)

Question 3. sin (ax + b)

Question 4. sec (tan ( x ))

Question 5. sin ( ) cos ( ) ax b cx d + +

Question 6. cos x3 . sin2 (x5)

Question 7. 2 cot ( x2 )

Question 8. cos( x )

Question 9. Prove that the function f given by f (x) = | x – 1 |, x ∈ R is not differentiable at x = 1.

Question 10. Prove that the greatest integer function defined by f (x) = [x], 0 < x < 3 is not differentiable at x = 1 and x = 2.

EXERCISE 5.4

Differentiate the following w.r.t. x:

Question 1. sin ex x

Question 2. esin 1 x −

Question 3. ex3

Question 4. sin (tan–1 e–x)

Question 5. log (cos ex)

Question 6. 2 5 ex + ex +... + ex

Question 7. e x , x > 0

Question 8. log (log x), x > 1

Question 9. cos , 0 log x x x >

Question 10. cos (log x + ex), x > 0

EXERCISE 5.5

Differentiate the functions given in Exercises 1 to 11 w.r.t. x.

Question 1. cos x . cos 2x . cos 3x

Question 2. ( 1)( 2) ( 3)( 4)( 5) x x x x x − − − − −

Question 3. (log x)cos x

Question 4. xx – 2sin x

Question 5. (x + 3)2 . (x + 4)3 . (x + 5)4

Question 6. 1 1 1

Question 7. (log x)x + xlog x

Question 8. (sin x)x + sin–1 x

Question 9. xsin x + (sin x)cos x

Question 10. 2 cos 2 1 1 xx x x x + + −

Question 11. (x cos x)x + 1 (xsin x)x Find dy dx of the functions given in Exercises 12 to 15.

Question 12. xy + yx = 1

Question 13. yx = xy

Question 14. (cos x)y = (cos y)x

Question 15. xy = e(x – y)

Question 16. Find the derivative of the function given by f (x) = (1 + x) (1 + x2) (1 + x4) (1 + x8) and hence find f ′(1).

Question 17. Differentiate (x2 – 5x + 8) (x3 + 7x + 9) in three ways mentioned below:
(i) by using product rule
(ii) by expanding the product to obtain a single polynomial.
(iii) by logarithmic differentiation. Do they all give the same answer?

Question 18. If u, v and w are functions of x, then show that d dx (u. v. w) = du dx v. w + u . dv dx . w + u . v dw dx in two ways - first by repeated application of product rule, second by logarithmic differentiation.

EXERCISE 5.6

If x and y are connected parametrically by the equations given in Exercises 1 to 10, without eliminating the parameter, Find dy dx .

Question 1. x = 2at2, y = at4

Question 2. x = a cos θ, y = b cos θ

Question 3. x = sin t, y = cos 2t 4. x = 4t, y = 4 t

Question 5. x = cos θ – cos 2θ, y = sin θ – sin 2θ 6. x = a (θ – sin θ), y = a (1 + cos θ)

Question 7. x = sin3 cos 2 t t , cos3 cos2 y t t =

Question 8. cos log tan 2 x = a = a sin t

Question 9. x = a sec θ, y = b tan θ

Question 10. x = a (cos θ + θ sin θ), y = a (sin θ – θ cos θ)

Question 11. If x asin 1 t , y acos 1 t , show that dy y dx x

EXERCISE 5.7

Find the second order derivatives of the functions given in Exercises 1 to 10.

Question1. x2 + 3x +

Question 2. x20

Question 3. x . cos x

Question 4. log x

Question 5. x3 log x

Question 6. ex sin 5x

Question 7. e6x cos 3x

Question 8. tan–1 x

Question 9. log (log x)

Question 10. sin (log x)

Question 11. If y = 5 cos x – 3 sin x, prove that 2 2 d y y 0 dx + =

Question 12. If y = cos–1 x, Find 2 2 d y dx in terms of y alone.

Question 13. If y = 3 cos (log x) + 4 sin (log x), show that x2 y2 + xy1 + y = 0

Question 14. If y = Aemx + Benx, show that 2 2 d y (m n) dy mny 0 dx dx − + + =

Question 15. If y = 500e7x + 600e– 7x, show that 2 2 d y 49y dx =

Question 16. If ey (x + 1) = 1, show that 2 2 2 d y dy dx dx =

Question 17. If y = (tan–1 x)2, show that (x2 + 1)2 y2 + 2x (x2 + 1) y1 = 2

Please Wait pdf file is loading (कृपया इंतजार करें pdf file लोड हो रही है)...
Loading speed will depend up on your download speed. Pdf file के लोड होने में लगा समय आपकी डाउनलोड स्पीड पर निर्भर करेगा

Download pdf file links for Continuity and Differentiability Class 12 NCERT Solutions

Ncert Solution for class 6 to 12 download in pdf

Follow Us On

Facebook

Twitter

Youtube

Linkdin